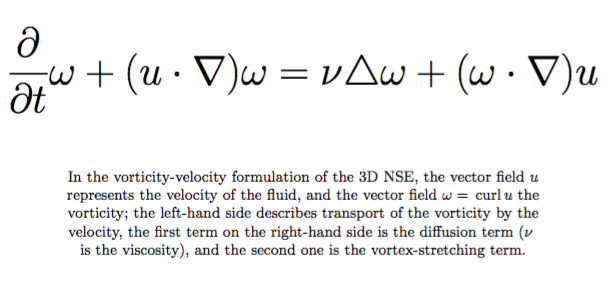Consent Form for ScienceAtHome user accounts.
1. Project title
In connection to the research project ScienceAtHome will we need your consent to process your personal data in accordance with the data protection regulation.
2. Project description
We, researchers from ScienceAtHome, Aarhus University, Ny Munkegade 120, 8000 Århus C, Den-mark are inviting you to participate in our research by using ScienceAtHome tools and games. By using the ScienceAtHome tools and games, you are participating in the global movement to break down the barriers between researchers and the general public and in the pursuit of a world in which every citizen is given equal opportunity to thrive in an increasingly digitized world. Research in natural sciences within ScienceAtHome will help researchers address large outstanding challenges like the development of a quantum computer and the understanding of the processes underlying turbulent flows as well as improving tools for teaching in high schools and universities. Research in social sciences helps us understand reasoning and problem solving from the perspective of psychology and behavioural economics. Additionally, we will develop and give you a player profile based on your performance on the ScienceAtHome games and tools.
Your account is saved in a database and used to link the data collected from our different projects together, so we can perform cross-correlation studies of demographic effects upon in-game behaviour. Your personal data; email, name, and address will only be used to verify that you are a real person, enable you to access your account and its accompanying information, enable you to make queries about the data you have generated in the game and to allow us to communicate with you.
3. Data responsible, project group, and project leader
Aarhus University, CVR nr. 31119103, is Data responsible for the processing of your personal data. Sci-enceAtHome is responsible for the project. ScienceAtHome is lead by Jacob Sherson and can be contacted on Ny Munkegade 120, 8000 Aarhus C, director@scienceathome.org
ScienceAtHome is an interdisciplinary team of scientists, game developers, designers, and visual artists employed at Aarhus University, Department of Physics and Astronomy, Denmark. We create fun scientific games, with the aim of revolutionizing scientific research and teaching by game-play.
4. Categories of personal data that will be processed about you
We process regular personal data in the form of email, name, nickname, gender, date of birth, nationality, city and country of residence, educational level, field of employment/study, IP-address, and user-generated data.
5. Purpose and processing activities
We store your personal data (email, name, nickname, gender, date of birth, nationality, city and country of residence, educational level, field of employment/study, IP-address, and user-generated data) for the purpose of developing a quantum computer and optimisation algorithms, improving tools for teaching in high schools and universities, as well as validating cognitive models. User-generated data are only recorded from your actions within the application.
The nickname you choose for your ScienceAtHome account will be used to show your ranking on leaderboards in the different games and would be seen by other ScienceAtHome users. The leaderboards will be displayed in the respective games on which they are based. In connection with competitions and events, the leaderboards are made available on our website for ScienceAtHome users. In some cases, the leaderboards are made publicly available on our website.
In connection with your use of the tools and games, we may send you service announcements, administrative messages, and other information via e-mail. The research team at ScienceAtHome might use this contact information for asking questions about our games and tools in order to improve your experience. It is not mandatory to reply to these emails and you can opt out of some or all of those communications. In case of a need for sharing your personal data with a third party or processing your data for other research purposes, we will contact you regarding a new consent form.
6. Confidentiality
Statistical procedures including the pseudonymization of data are followed at all times during data processing. In case personally identifiable data are required, (for example, for creating your personal gaming profile) exception can be made under the guidelines of ScienceAtHome’s ethical committee. We guarantee that identifiers are not released during or after the research study to anyone except the direct research team. Your participation in the study is strictly confidential.
7. Time of storage
Your personally identifiable information is stored for two years from your last activity on our services. If our system discovers that your account has been inactive for more than two years, you will be contacted and given a notice before your account is deleted, and the data are anonymized.
8. Age of participation
The EU GDPR 2016/679 only allows us to gather and store information from people below the age of 16 years with the explicit consent of their parents; therefore, people below 16 years old are not allowed to sign up to our ScienceAtHome platform or any of the games or tools. If you are below 16 years old and want to participate in our studies or use our tools, you can ask your parents to contact us and ask us about how to participate.
9. Queries
In case you have any questions about how we use your data, you can contact us to inquire more about it or to request the anonymization of your entries in the database. Please do so by emailing: datamanager@scienceathome.org.
However, if you require your data to be anonymized, all your game profiles and progress in games will be lost; and your user account will be revoked.
10. Withdraw consent
Participation is voluntary and you can at any time withdraw your consent to the processing of personal information. You can do this by sending an email to datamanager@scienceathome.org form the same email as you have registered the account. If you withdraw your consent, then it will only be in effect from this time, and it will not affect the legality of the processing up until that time.
11. Consent
1. I confirm that I have read and understood the information presented to me in this document. I have had the opportunity to consider the information, ask questions and have had this answered satisfactorily.
2. I understand that my participation is voluntary and that I am free to withdraw my consent at any time without giving any reason.
3. I am in control of any kind of linking or data sharing from my ScienceAtHome account to any social networks. This responsibility is not linked to the research team or the research centre.
4. I am 16 years old or older
By accepting this consent form, I confirm that I have read and agree to the terms and conditions and that ScienceAtHome may process my personal data from the following games:
• Alien Code: Discover the pattern behind the Alien Code and help us learn about human problem-solving.
• Crystal Crop Fever: Collaboratively explore the landscapes and help us study the power of team-work.
• Diff Tool: A tool that helps you conceptualize the principles of differentiation.
• Inequality Game: Evaluate and distribute the resources and help us understand the strategies in socioeconomic models.
• Netty: A game to explore human solutions to NP-hard problems in computer science.
• Skill Lab: Science Detective: Find out your cognitive profile by playing a collection of mini-games.
• Potential Penguin: Explore the connections between potential and kinetic energy, in a fun educational game.
• Quantum Carnival: Go to the carnival and learn how to control the atoms.
• Quantum Dreams: Glide through the worlds of classical computers chasing the dream of quantum computing.
• Quantum Moves: Help us build a quantum computer by transporting atoms and contribute to cutting-edge physics.
• Quantum Minds: Transport the atoms and help us understand how to build a quantum intuition.
• Quantum Shooter: Defend yourself against the enemies, load your weapons with atoms
• Stat World: Explore the power of statistics in a playful environment.
• Tower Builder: Build the tower and learn something about your own skills.
• Turbulence: Find the largest air pockets and join our quest to unlock the mysteries of the “most important unsolved problem in classical physics”.
And for the purposes of